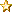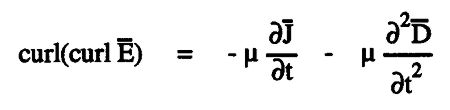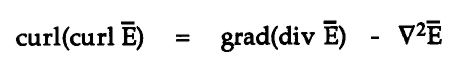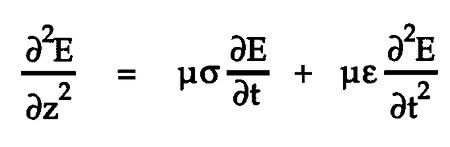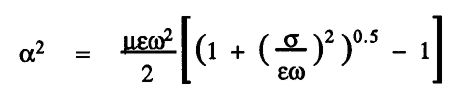Maxwell's equations support a wave equation that governs the propagation of both the electric and magnetic fields in space and time, where the wave equation describing a propagating electric field E-Bar in a general lossy medium of conductivity ? (sigma), permittivity ? (epsilon) and permeability µ (mu) can be succinctly derived as follows:
Applying the vector operator curl on the Faraday equation,
Substituting, B-Bar = µH-Bar and for curl H-Bar from Ampere's law,
Substituting J-Bar = ? E-Bar , D-Bar = ?bE-Bar and using the vector identity,
The generalized wave equation in a conductive medium then follows as
In this equation, ?2 is the vector Laplace operator, and we have assumed from Gauss's theorem that div bE-Bar = r/? = 0 for a charge-free region. A similar equation can also be derived in terms of the H-Bar field, where, because of the symmetry of Maxwell's equations,
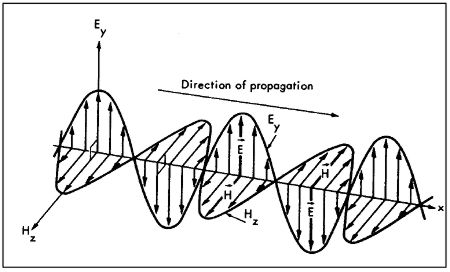
In practice we shall consider only the E-Bar field, as the H-Bar field can be derived from Faraday's law by integrating over time the vector curl E-Bar, which reveals that at every point in space E-Bar and H-Bar are mutually at right angles, and also lie in a plane at right angles to the direction of propagation (fig.1).
Fig.1 A propagating electromagnetic wave. The sinusoidally varying magnetic field is at right angles to the sinusoidally varying electric field, and both are at right angles to the direction of propagation.
Consider a steady-state, sinusoidal electric field E-Bar propagating within a medium of finite conductivity where, because of the conversion of electrical energy into heat within a conductor, the traveling wave must experience attenuation. This suggests that a steady-state wave of sinusoidal form should decay exponentially as a function of distance z,
E = E??zsin(?t ?z)
? (alpha) is defined as the attenuation constant, while the phase of the wave as a function of distance is determined by the phase constant ? (beta) = 2?/?, where ? (in meters) is the wavelength of the propagating field and ? (omega) = 2?f, the angular frequency in radians/second.
An exponential decay is a logical choice, as for each unit distance the wave propagates it is attenuated by the same fractional amount. The electric field E is aligned to propagate in a direction z, where the direction of E is at 90° (right-angles) to z as shown in fig.1, where several phases are illustrated. Consequently, at a fixed point of observation z, E varies sinusoidally, while for constant time t, E plotted against z is a sinewave with exponential decay.
To check the validity of this solution, the function for E must satisfy the wave equation. This validation also enables the constants ? and ? to be expressed as functions of ?, ?, µ, and ?. However, because this substitution, although straightforward, is somewhat tedious, I will show only the initial working and then state the conclusion:
Substitute the assumed solution into the wave equation where, if propagation is assumed to take the direction z,
It follows that the function for E is a solution to the wave equation, provided that
?2 ?2 = µ??2
?? = ?µ?/2
Hence solving for ? and ?,
? = ?µ?/2?
where the constants ? and ? that govern the velocity and attenuation of the propagating field can be expressed in terms of the angular frequency ? and the parameters µ, ?, and ?, which are documented for most materials. (? and ? are sometimes expressed as a complex number in terms of the propagation constant ? (gamma), where ? = ? + j?.)